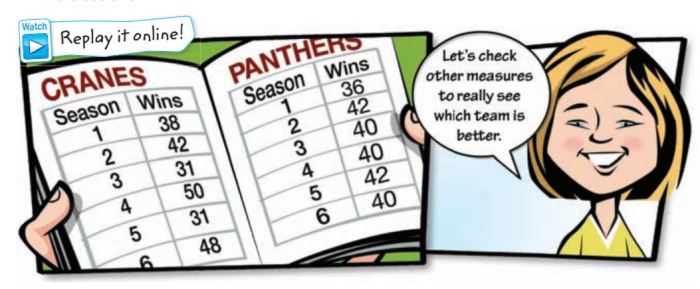
Course 1 Chapter 11 Statistical Measures Answer Key: Unlocking Data Insights introduces a comprehensive understanding of statistical measures, their significance, and their applications in various fields. This guide provides clear explanations, engaging examples, and insightful discussions to empower readers with the knowledge and skills to analyze and interpret data effectively.
Statistical measures are essential tools for understanding the patterns, trends, and relationships within data. They help researchers, analysts, and decision-makers summarize, describe, and draw meaningful conclusions from complex datasets. This chapter explores a wide range of statistical measures, including measures of central tendency, dispersion, shape, and relationship, equipping readers with the knowledge to handle data with confidence and accuracy.
Statistical Measures Overview: Course 1 Chapter 11 Statistical Measures Answer Key

Statistical measures are tools that help us understand and describe data. They provide numerical summaries of various aspects of data, enabling us to make inferences and draw conclusions about the underlying population. Statistical measures are widely used in various fields, including research, business, finance, and social sciences.
Examples of common statistical measures include:
- Measures of central tendency (mean, median, mode)
- Measures of dispersion (range, variance, standard deviation)
- Measures of shape (skewness, kurtosis)
- Measures of relationship (correlation, regression)
Measures of Central Tendency, Course 1 chapter 11 statistical measures answer key
Measures of central tendency provide a single value that represents the “center” of a dataset. They are useful for comparing different datasets and understanding the typical value of a variable.
The most common measures of central tendency are:
- Mean:The average of all values in a dataset.
- Median:The middle value of a dataset when arranged in ascending order.
- Mode:The value that occurs most frequently in a dataset.
Each measure has its advantages and disadvantages, as summarized in the following table:
| Measure | Advantages | Disadvantages |
|---|---|---|
| Mean | Most widely used, provides a stable estimate | Sensitive to outliers, can be misleading with skewed data |
| Median | Less sensitive to outliers, more robust | Not as efficient as mean, can be unstable with small sample sizes |
| Mode | Easy to understand, represents the most common value | Not always unique, can be misleading with multimodal data |
Measures of Dispersion
Measures of dispersion describe how spread out a dataset is. They are useful for understanding the variability of a variable and identifying outliers.
Common measures of dispersion include:
- Range:The difference between the maximum and minimum values in a dataset.
- Variance:The average of the squared differences between each value and the mean.
- Standard deviation:The square root of the variance.
The following table provides the formulas and interpretations for these measures:
| Measure | Formula | Interpretation |
|---|---|---|
| Range | Maximum
|
Overall spread of the data |
| Variance | Σ(x
|
Average squared deviation from the mean |
| Standard deviation | √Variance | Typical deviation from the mean |
Essential FAQs
What is the purpose of statistical measures?
Statistical measures provide a concise and informative summary of data, allowing researchers to describe, analyze, and draw meaningful conclusions from complex datasets.
What are the different types of statistical measures?
Statistical measures can be categorized into measures of central tendency (e.g., mean, median, mode), measures of dispersion (e.g., range, variance, standard deviation), measures of shape (e.g., skewness, kurtosis), and measures of relationship (e.g., correlation, regression).
How are statistical measures used in real-world applications?
Statistical measures are widely used in various fields, including healthcare, finance, marketing, and social sciences. They help researchers analyze data, identify trends, make predictions, and inform decision-making.